
These complex amplitude vectors are not functions of time, as they are understood to refer to oscillations over all time. We would thus not be considering the instantaneous E( t) and H( t) used above, but rather a complex (vector) amplitude for each which describes a coherent wave's phase (as well as amplitude) using phasor notation. The results can then be applied more generally, for instance, by representing incoherent radiation as a superposition of such waves at different frequencies and with fluctuating amplitudes. More commonly, problems in electromagnetics are solved in terms of sinusoidally varying fields at a specified frequency. The above form for the Poynting vector represents the instantaneous power flow due to instantaneous electric and magnetic fields. Equation 1 In Equation 1, is the permittivity of the medium (material) where we are measuring the fields. The Poynting vector and theorem and expression for energy density are universally valid in vacuum and all materials. Since only the microscopic fields E and B occur in the derivation of S = (1/ μ 0) E × B and the energy density, assumptions about any material present are avoided. In all other cases, they differ in that S = (1/ μ 0) E × B and the corresponding u are purely radiative, since the dissipation term − J ⋅ E covers the total current, while the E × H definition has contributions from bound currents which are then excluded from the dissipation term. The two alternative definitions of the Poynting vector are equal in vacuum or in non-magnetic materials, where B = μ 0 H. It can be derived directly from Maxwell's equations in terms of total charge and current and the Lorentz force law only.
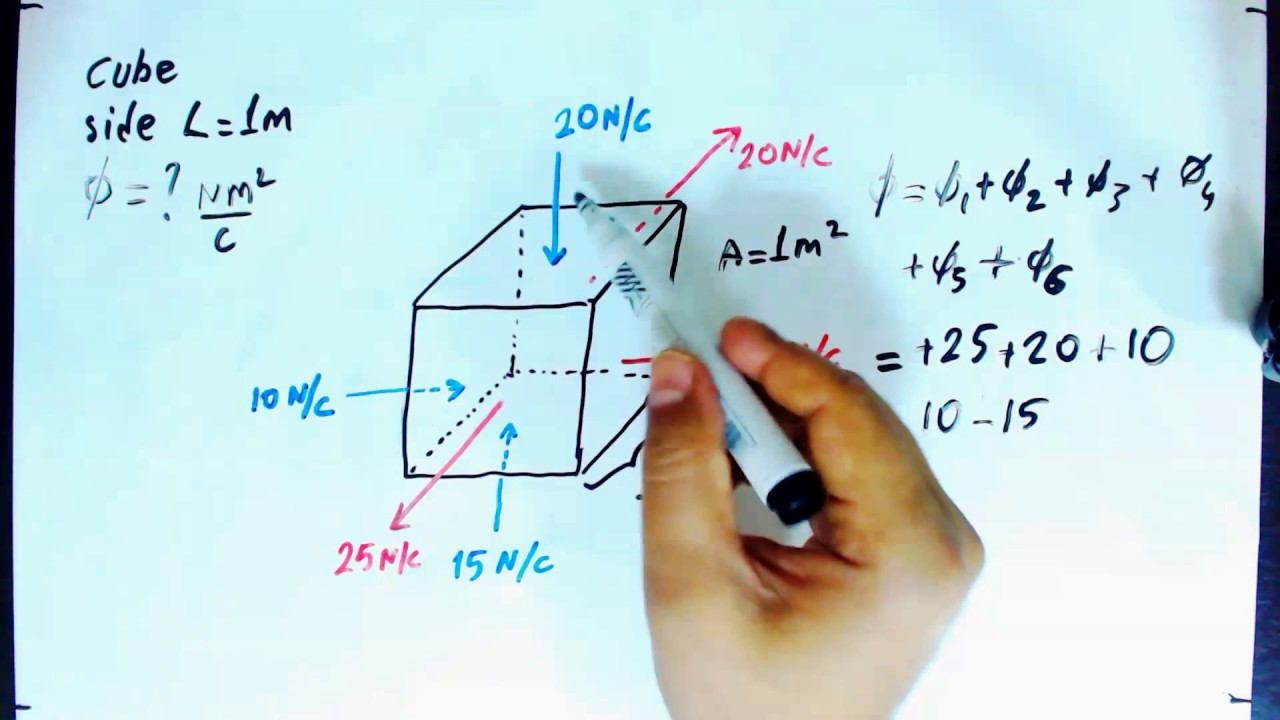
The electric flux E is then defined as a surface integral of the electric field.

In Poynting's original paper and in most textbooks, the Poynting vector S Gauss’s law 0 E Q/0 We calculate the electric flux through each element and integrate the results to obtain the total flux. The Poynting vector is used throughout electromagnetics in conjunction with Poynting's theorem, the continuity equation expressing conservation of electromagnetic energy, to calculate the power flow in electromagnetic fields. Oliver Heaviside also discovered it independently in the more general form that recognises the freedom of adding the curl of an arbitrary vector field to the definition. : 132 Nikolay Umov is also credited with formulating the concept. It is named after its discoverer John Henry Poynting who first derived it in 1884. The SI unit of the Poynting vector is the watt per square metre (W/m 2) kg/s 3 in base SI units. Now that we have defined the area vector of a surface, we can define the electric flux of a uniform electric field through a flat area as the scalar product of the electric field and the area vector, as defined in Products of Vectors: E A(uniformE, flat surface).

What is the electric flux Answer: From the. In physics, the Poynting vector (or Umov–Poynting vector) represents the directional energy flux (the energy transfer per unit area per unit time) or power flow of an electromagnetic field. 1) A planar surface has an area of 1 m2, if an electric field crosses with an angle of 30 to it, and has E 2 V/m.


 0 kommentar(er)
0 kommentar(er)
